Bodensee
Question
Solution
Short
Video
\(\LaTeX\)
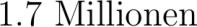
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
In einem Gedankenexperiment entimmt man dem Bodensee pqkm^ einen Teelöffel Wasser pqcm^ und markiert die so geschöpften Moleküle rot und giesst dann das Wasser in den See zurück. Die rot markierten Moleküle verteilen sich nun gleichmässig im See. Wie viele dieser Moleküle erhält man wieder wenn man zum zweitenmal einen Teelöffel Wasser schöpft?
Solution:
Für den Teelöffel gelten folge Daten: V_E pqcm^ pqm^ m_E pqkgpmk pqm^ pq.kg pqg N_E fracm_EM pq.emol^- numpr.e. Wenn ein Teelöffel dem Bodensee entnommen wird und alle Moleküle rot markiert werden in Realität natürlich nicht zu realisieren so werden anschliess numpr.e rote Moleküle im Bodensee verteilt rumschwimmen. Für den Bodensee gilt mit den angegebenen Daten: V_O pqkm^ pqem^ pq.em^ m_O pqkgpmk pq.em^ pq.ekg N_O fracm_OM pq.emol^- numpr.e. Das ganze Bodenseewasser besteht laut dieser Rechnung aus numpr.e Wassermolekülen. Jedes fracN_ON_E numpr.e-mboxte Wassermolekül im Bodensee ist rot gefärbt. Wenn jedes numpr.e-te Molekül im Bodensee rot ist so ist auch jedes numpr.e-te Molekül in einem beliebigen Teelöffel Wasser den wir irgwo dem Bodensee entnehmen rot gefärbt. D.h. im Mittel werden etwa numpr.e gerundet . Millionen rot gefärbte Moleküle in jedem Teelöffel Wasser wiedergefunden.
In einem Gedankenexperiment entimmt man dem Bodensee pqkm^ einen Teelöffel Wasser pqcm^ und markiert die so geschöpften Moleküle rot und giesst dann das Wasser in den See zurück. Die rot markierten Moleküle verteilen sich nun gleichmässig im See. Wie viele dieser Moleküle erhält man wieder wenn man zum zweitenmal einen Teelöffel Wasser schöpft?
Solution:
Für den Teelöffel gelten folge Daten: V_E pqcm^ pqm^ m_E pqkgpmk pqm^ pq.kg pqg N_E fracm_EM pq.emol^- numpr.e. Wenn ein Teelöffel dem Bodensee entnommen wird und alle Moleküle rot markiert werden in Realität natürlich nicht zu realisieren so werden anschliess numpr.e rote Moleküle im Bodensee verteilt rumschwimmen. Für den Bodensee gilt mit den angegebenen Daten: V_O pqkm^ pqem^ pq.em^ m_O pqkgpmk pq.em^ pq.ekg N_O fracm_OM pq.emol^- numpr.e. Das ganze Bodenseewasser besteht laut dieser Rechnung aus numpr.e Wassermolekülen. Jedes fracN_ON_E numpr.e-mboxte Wassermolekül im Bodensee ist rot gefärbt. Wenn jedes numpr.e-te Molekül im Bodensee rot ist so ist auch jedes numpr.e-te Molekül in einem beliebigen Teelöffel Wasser den wir irgwo dem Bodensee entnehmen rot gefärbt. D.h. im Mittel werden etwa numpr.e gerundet . Millionen rot gefärbte Moleküle in jedem Teelöffel Wasser wiedergefunden.