Landendes Flugzeug
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

Need help? Yes, please!
The following quantities appear in the problem:
Masse \(m\) / Kraft \(F\) / Geschwindigkeit \(v\) / Strecke \(s\) / Beschleunigung \(a\) /
The following formulas must be used to solve the exercise:
\(s = \dfrac{1}{2}at^2+v_0 t \quad \) \(F = ma \quad \)

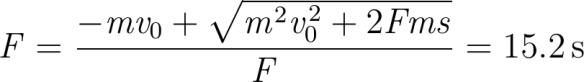
No explanation / solution video for this exercise has yet been created.
But there is a video to a similar exercise:
In case your browser prevents YouTube embedding: https://youtu.be/Lvt0UzOx3s8
But there is a video to a similar exercise:
Exercise:
Ein Flugzeug mit t Masse landet mit kilometerperhour und erfährt dann kN bremse Kraft. Wie lange braucht das Flugzeug für den ersten Kilometer auf der Landebahn?
Solution:
Geg m t .ekg v_ . F -kN -.eN s km m % GesZeitt sis % Das Flugzeug erfährt eine Bremsbeschleunigung von al a fracFm frac-.eN.ekg -.meterpersecondsquared. Die Position s eines gleichmässig mit a beschleunigten Körpers bei gegebener Anfangsgeschwindigkeit v_ ist als v_ t + fracat^. Setzen wir für die Beschleunigung afracFm ein so erhalten wir als v_ t + fracFm t^. Wir lösen diese Gleichung nach der Zeit auf: al s v_ t + fracFm t^ uf -s fracFmt^ + v_ t - s uf m fracFt^ + mv_t - ms mf t frac-mv_ + sqrtm^v_^ - qtyfracF -ms fracF frac-mv_ + sqrtm^v_^ + FmsF t_ .s t_ .s Das Flugzeug benötigt also gut Sekunden für den ersten Kilometer. % t frac-mv_ + sqrtm^v_^ + FmsF .s
Ein Flugzeug mit t Masse landet mit kilometerperhour und erfährt dann kN bremse Kraft. Wie lange braucht das Flugzeug für den ersten Kilometer auf der Landebahn?
Solution:
Geg m t .ekg v_ . F -kN -.eN s km m % GesZeitt sis % Das Flugzeug erfährt eine Bremsbeschleunigung von al a fracFm frac-.eN.ekg -.meterpersecondsquared. Die Position s eines gleichmässig mit a beschleunigten Körpers bei gegebener Anfangsgeschwindigkeit v_ ist als v_ t + fracat^. Setzen wir für die Beschleunigung afracFm ein so erhalten wir als v_ t + fracFm t^. Wir lösen diese Gleichung nach der Zeit auf: al s v_ t + fracFm t^ uf -s fracFmt^ + v_ t - s uf m fracFt^ + mv_t - ms mf t frac-mv_ + sqrtm^v_^ - qtyfracF -ms fracF frac-mv_ + sqrtm^v_^ + FmsF t_ .s t_ .s Das Flugzeug benötigt also gut Sekunden für den ersten Kilometer. % t frac-mv_ + sqrtm^v_^ + FmsF .s
Meta Information
Exercise:
Ein Flugzeug mit t Masse landet mit kilometerperhour und erfährt dann kN bremse Kraft. Wie lange braucht das Flugzeug für den ersten Kilometer auf der Landebahn?
Solution:
Geg m t .ekg v_ . F -kN -.eN s km m % GesZeitt sis % Das Flugzeug erfährt eine Bremsbeschleunigung von al a fracFm frac-.eN.ekg -.meterpersecondsquared. Die Position s eines gleichmässig mit a beschleunigten Körpers bei gegebener Anfangsgeschwindigkeit v_ ist als v_ t + fracat^. Setzen wir für die Beschleunigung afracFm ein so erhalten wir als v_ t + fracFm t^. Wir lösen diese Gleichung nach der Zeit auf: al s v_ t + fracFm t^ uf -s fracFmt^ + v_ t - s uf m fracFt^ + mv_t - ms mf t frac-mv_ + sqrtm^v_^ - qtyfracF -ms fracF frac-mv_ + sqrtm^v_^ + FmsF t_ .s t_ .s Das Flugzeug benötigt also gut Sekunden für den ersten Kilometer. % t frac-mv_ + sqrtm^v_^ + FmsF .s
Ein Flugzeug mit t Masse landet mit kilometerperhour und erfährt dann kN bremse Kraft. Wie lange braucht das Flugzeug für den ersten Kilometer auf der Landebahn?
Solution:
Geg m t .ekg v_ . F -kN -.eN s km m % GesZeitt sis % Das Flugzeug erfährt eine Bremsbeschleunigung von al a fracFm frac-.eN.ekg -.meterpersecondsquared. Die Position s eines gleichmässig mit a beschleunigten Körpers bei gegebener Anfangsgeschwindigkeit v_ ist als v_ t + fracat^. Setzen wir für die Beschleunigung afracFm ein so erhalten wir als v_ t + fracFm t^. Wir lösen diese Gleichung nach der Zeit auf: al s v_ t + fracFm t^ uf -s fracFmt^ + v_ t - s uf m fracFt^ + mv_t - ms mf t frac-mv_ + sqrtm^v_^ - qtyfracF -ms fracF frac-mv_ + sqrtm^v_^ + FmsF t_ .s t_ .s Das Flugzeug benötigt also gut Sekunden für den ersten Kilometer. % t frac-mv_ + sqrtm^v_^ + FmsF .s
Contained in these collections:
-
Newton II by aej
-
Aktionsprinzip by pw
-
Kraftwirkungsgesetz by uz
-
Lex Secunda und 1. Formel by TeXercises
Asked Quantity:
Zeit \(t\)
in
Sekunde \(\rm s\)
Physical Quantity
Die Zeit beschreibt die Abfolge von Ereignissen, hat also eine eindeutige, nicht umkehrbare Richtung.
Unit
Seit 1967 ist eine Sekunde das 9.192.631.770-fache der Periodendauer der Strahlung, die dem Übergang zwischen den beiden Hyperfeinstrukturniveaus des Grundzustandes von Atomen des Nuklids 133Cs entspricht.
Base?
SI?
Metric?
Coherent?
Imperial?

