Masseloser Holzstab als zweiseitiger Hebel
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

Need help? Yes, please!
The following quantities appear in the problem:
Länge \(\ell\) / Kraft \(F\) / Drehmoment \(\vec M\) /
The following formulas must be used to solve the exercise:
\(\vec M = \vec \ell \times \vec F \quad \) \(\sum \stackrel{\curvearrowleft}{M} \stackrel{!}{=} \sum \stackrel{\curvearrowright}{M} \quad \)

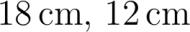
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
Gegeben sei ein .cm langer Holzstab welcher als masselos betrachtet werden darf. Wo muss er aufgehängt werden damit er sich im Gleichgewicht befindet wenn man an das eine Ende g und an das andere Ende g hängt?
Solution:
newqtylcm newqtymeg newqtymzg % Die beiden hervorgerufenen Drehmomente müssen sich das Gleichgewicht halten d.h. es gilt das glqq Hebelgesetzgrqq: DrehmomentSchritte PGleichung stackrelcurvearrowleftM stackrelcurvearrowrightM PGleichungM_ M_ PGleichungF_ell_ F_ell_ PGleichungm_gell_ m_g ell-ell_ MGleichungm_gell_ m_g ell-m_gell_ MGleichungm_gell_+m_gell_ m_g ell MGleichungm_+m_gell_ m_g ell MGleichungell_ fracm_ ellm_+m_ % PHYSMATH % Der erste Hebelarm ist somit solqtylefracm_ ellm_+m_mzn*ln/men+mzncm al ell_ lef fracmzlme+mz leTT Für den zweiten Hebelarm folgt solqtylzfracm_ellm_+m_ln-lencm al ell_ ell - ell_ lzf l - leTT lzTT. Die Aufgabe kann selbstverständlich auch mit Verhältnissen gelöst werden; der Hebel ist in Teile zu unterteilen da Newton totale Kraft. Die Hebelarme sind dann entsprech dem Verhältnis : den Kräften zuzuordnen.
Gegeben sei ein .cm langer Holzstab welcher als masselos betrachtet werden darf. Wo muss er aufgehängt werden damit er sich im Gleichgewicht befindet wenn man an das eine Ende g und an das andere Ende g hängt?
Solution:
newqtylcm newqtymeg newqtymzg % Die beiden hervorgerufenen Drehmomente müssen sich das Gleichgewicht halten d.h. es gilt das glqq Hebelgesetzgrqq: DrehmomentSchritte PGleichung stackrelcurvearrowleftM stackrelcurvearrowrightM PGleichungM_ M_ PGleichungF_ell_ F_ell_ PGleichungm_gell_ m_g ell-ell_ MGleichungm_gell_ m_g ell-m_gell_ MGleichungm_gell_+m_gell_ m_g ell MGleichungm_+m_gell_ m_g ell MGleichungell_ fracm_ ellm_+m_ % PHYSMATH % Der erste Hebelarm ist somit solqtylefracm_ ellm_+m_mzn*ln/men+mzncm al ell_ lef fracmzlme+mz leTT Für den zweiten Hebelarm folgt solqtylzfracm_ellm_+m_ln-lencm al ell_ ell - ell_ lzf l - leTT lzTT. Die Aufgabe kann selbstverständlich auch mit Verhältnissen gelöst werden; der Hebel ist in Teile zu unterteilen da Newton totale Kraft. Die Hebelarme sind dann entsprech dem Verhältnis : den Kräften zuzuordnen.
Meta Information
Exercise:
Gegeben sei ein .cm langer Holzstab welcher als masselos betrachtet werden darf. Wo muss er aufgehängt werden damit er sich im Gleichgewicht befindet wenn man an das eine Ende g und an das andere Ende g hängt?
Solution:
newqtylcm newqtymeg newqtymzg % Die beiden hervorgerufenen Drehmomente müssen sich das Gleichgewicht halten d.h. es gilt das glqq Hebelgesetzgrqq: DrehmomentSchritte PGleichung stackrelcurvearrowleftM stackrelcurvearrowrightM PGleichungM_ M_ PGleichungF_ell_ F_ell_ PGleichungm_gell_ m_g ell-ell_ MGleichungm_gell_ m_g ell-m_gell_ MGleichungm_gell_+m_gell_ m_g ell MGleichungm_+m_gell_ m_g ell MGleichungell_ fracm_ ellm_+m_ % PHYSMATH % Der erste Hebelarm ist somit solqtylefracm_ ellm_+m_mzn*ln/men+mzncm al ell_ lef fracmzlme+mz leTT Für den zweiten Hebelarm folgt solqtylzfracm_ellm_+m_ln-lencm al ell_ ell - ell_ lzf l - leTT lzTT. Die Aufgabe kann selbstverständlich auch mit Verhältnissen gelöst werden; der Hebel ist in Teile zu unterteilen da Newton totale Kraft. Die Hebelarme sind dann entsprech dem Verhältnis : den Kräften zuzuordnen.
Gegeben sei ein .cm langer Holzstab welcher als masselos betrachtet werden darf. Wo muss er aufgehängt werden damit er sich im Gleichgewicht befindet wenn man an das eine Ende g und an das andere Ende g hängt?
Solution:
newqtylcm newqtymeg newqtymzg % Die beiden hervorgerufenen Drehmomente müssen sich das Gleichgewicht halten d.h. es gilt das glqq Hebelgesetzgrqq: DrehmomentSchritte PGleichung stackrelcurvearrowleftM stackrelcurvearrowrightM PGleichungM_ M_ PGleichungF_ell_ F_ell_ PGleichungm_gell_ m_g ell-ell_ MGleichungm_gell_ m_g ell-m_gell_ MGleichungm_gell_+m_gell_ m_g ell MGleichungm_+m_gell_ m_g ell MGleichungell_ fracm_ ellm_+m_ % PHYSMATH % Der erste Hebelarm ist somit solqtylefracm_ ellm_+m_mzn*ln/men+mzncm al ell_ lef fracmzlme+mz leTT Für den zweiten Hebelarm folgt solqtylzfracm_ellm_+m_ln-lencm al ell_ ell - ell_ lzf l - leTT lzTT. Die Aufgabe kann selbstverständlich auch mit Verhältnissen gelöst werden; der Hebel ist in Teile zu unterteilen da Newton totale Kraft. Die Hebelarme sind dann entsprech dem Verhältnis : den Kräften zuzuordnen.
Contained in these collections:
-
Hebel I by pw
-
Holzstab als Hebel by TeXercises

