Schaltung
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

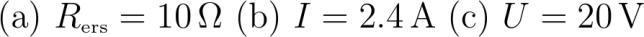
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
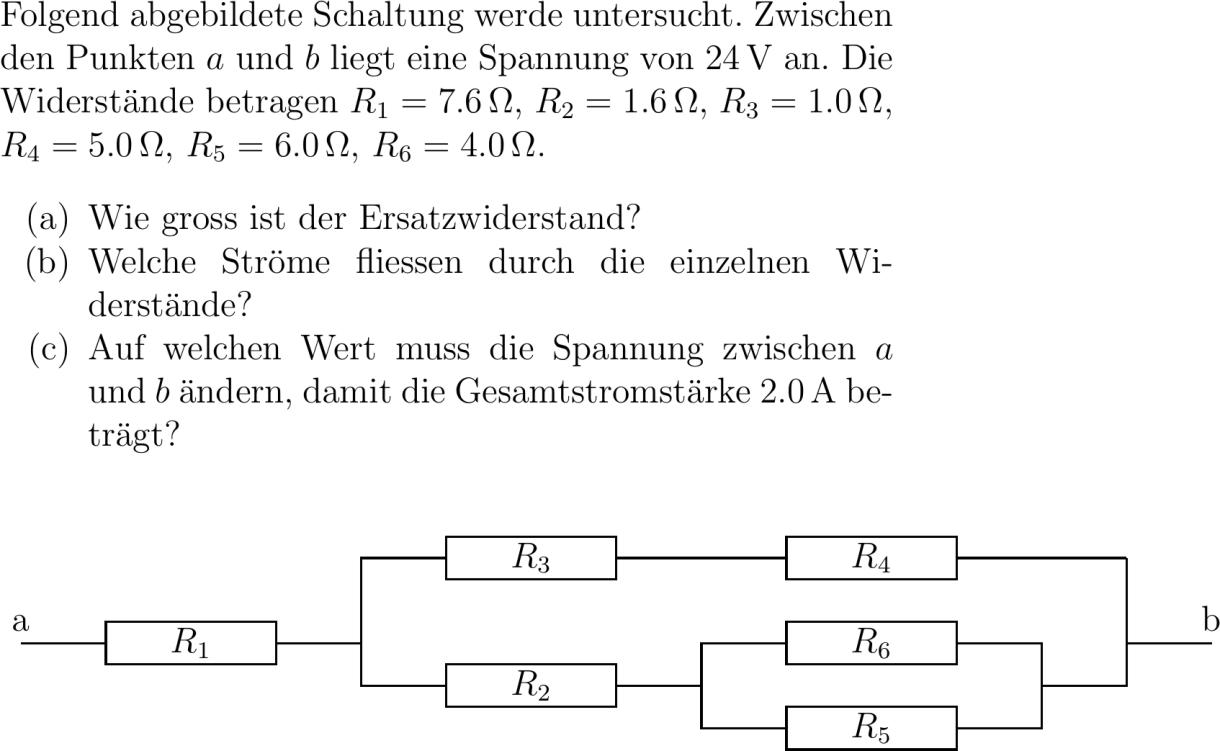
Folg abgebildete Schaltung werde untersucht. Zwischen den Punkten a und b liegt eine Spannung von pqV an. Die Widerstände betragen R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega. abcliste abc Wie gross ist der Ersatzwiderstand? abc Welche Ströme fliessen durch die einzelnen Widerstände? abc Auf welchen Wert muss die Spannung zwischen a und b ändern damit die Gesamtstromstärke pq.A beträgt? abcliste center tikzpicture drawthick ------; drawthick --; drawthick --; drawthick ----; drawthick ---.---.; drawthick -.---.----; drawthick -.------; drawthick -----.---.--; drawthick -------.; drawthick ----; nodeabove at - a; nodeabove at b; drawthick -. rectangle -.; drawthick . rectangle .; drawthick . rectangle .; drawthick -. rectangle -.; drawthick -. rectangle .; drawthick -. rectangle -.; node at R_; node at R_; node at -. R_; node at R_; node at - R_; node at - R_; tikzpicture center
Solution:
abcliste abc Der Ersatzwiderstand des oberen Stranges ist R_uparrow R_ + R_ pq.Omega . Der Ersatzwiderstand der Parallelwiderstände im unteren Strang ist R_downarrow parallel fracfracR_+fracR_ pq.Omega . Zusammen mit R_ macht das den unteren Strang R_downarrow R_ + R_downarrow parallel pq.Omega . Der untere und obere Strang sind parallel geschaltet ihr Ersatzwiderstand des rechten Teils ist damit R_rightarrow fracfracR_uparrow+fracR_downarrow pq.Omega . Zusammen mit dem Widerstand R_ in Serie gibt das dann RersR_+R_rightarrow pq.Omega. Das sind rund pqOmega. abc Über das Ohm'sche Gesetz können wir die totale Stromstärke ausrechnen die bei pqV durch die Schaltung fliesst I fracURers pq.A . Dieser Strom fliesst auch durch R_ also I_I. abc Falls nur pqA Totalstrom fliessen so liegen gemäss Ohm'schem Gesetz nur pqV an URers I. abcliste
Folg abgebildete Schaltung werde untersucht. Zwischen den Punkten a und b liegt eine Spannung von pqV an. Die Widerstände betragen R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega. abcliste abc Wie gross ist der Ersatzwiderstand? abc Welche Ströme fliessen durch die einzelnen Widerstände? abc Auf welchen Wert muss die Spannung zwischen a und b ändern damit die Gesamtstromstärke pq.A beträgt? abcliste center tikzpicture drawthick ------; drawthick --; drawthick --; drawthick ----; drawthick ---.---.; drawthick -.---.----; drawthick -.------; drawthick -----.---.--; drawthick -------.; drawthick ----; nodeabove at - a; nodeabove at b; drawthick -. rectangle -.; drawthick . rectangle .; drawthick . rectangle .; drawthick -. rectangle -.; drawthick -. rectangle .; drawthick -. rectangle -.; node at R_; node at R_; node at -. R_; node at R_; node at - R_; node at - R_; tikzpicture center
Solution:
abcliste abc Der Ersatzwiderstand des oberen Stranges ist R_uparrow R_ + R_ pq.Omega . Der Ersatzwiderstand der Parallelwiderstände im unteren Strang ist R_downarrow parallel fracfracR_+fracR_ pq.Omega . Zusammen mit R_ macht das den unteren Strang R_downarrow R_ + R_downarrow parallel pq.Omega . Der untere und obere Strang sind parallel geschaltet ihr Ersatzwiderstand des rechten Teils ist damit R_rightarrow fracfracR_uparrow+fracR_downarrow pq.Omega . Zusammen mit dem Widerstand R_ in Serie gibt das dann RersR_+R_rightarrow pq.Omega. Das sind rund pqOmega. abc Über das Ohm'sche Gesetz können wir die totale Stromstärke ausrechnen die bei pqV durch die Schaltung fliesst I fracURers pq.A . Dieser Strom fliesst auch durch R_ also I_I. abc Falls nur pqA Totalstrom fliessen so liegen gemäss Ohm'schem Gesetz nur pqV an URers I. abcliste
Meta Information
Exercise:
Folg abgebildete Schaltung werde untersucht. Zwischen den Punkten a und b liegt eine Spannung von pqV an. Die Widerstände betragen R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega. abcliste abc Wie gross ist der Ersatzwiderstand? abc Welche Ströme fliessen durch die einzelnen Widerstände? abc Auf welchen Wert muss die Spannung zwischen a und b ändern damit die Gesamtstromstärke pq.A beträgt? abcliste center tikzpicture drawthick ------; drawthick --; drawthick --; drawthick ----; drawthick ---.---.; drawthick -.---.----; drawthick -.------; drawthick -----.---.--; drawthick -------.; drawthick ----; nodeabove at - a; nodeabove at b; drawthick -. rectangle -.; drawthick . rectangle .; drawthick . rectangle .; drawthick -. rectangle -.; drawthick -. rectangle .; drawthick -. rectangle -.; node at R_; node at R_; node at -. R_; node at R_; node at - R_; node at - R_; tikzpicture center
Solution:
abcliste abc Der Ersatzwiderstand des oberen Stranges ist R_uparrow R_ + R_ pq.Omega . Der Ersatzwiderstand der Parallelwiderstände im unteren Strang ist R_downarrow parallel fracfracR_+fracR_ pq.Omega . Zusammen mit R_ macht das den unteren Strang R_downarrow R_ + R_downarrow parallel pq.Omega . Der untere und obere Strang sind parallel geschaltet ihr Ersatzwiderstand des rechten Teils ist damit R_rightarrow fracfracR_uparrow+fracR_downarrow pq.Omega . Zusammen mit dem Widerstand R_ in Serie gibt das dann RersR_+R_rightarrow pq.Omega. Das sind rund pqOmega. abc Über das Ohm'sche Gesetz können wir die totale Stromstärke ausrechnen die bei pqV durch die Schaltung fliesst I fracURers pq.A . Dieser Strom fliesst auch durch R_ also I_I. abc Falls nur pqA Totalstrom fliessen so liegen gemäss Ohm'schem Gesetz nur pqV an URers I. abcliste
Folg abgebildete Schaltung werde untersucht. Zwischen den Punkten a und b liegt eine Spannung von pqV an. Die Widerstände betragen R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega R_pq.Omega. abcliste abc Wie gross ist der Ersatzwiderstand? abc Welche Ströme fliessen durch die einzelnen Widerstände? abc Auf welchen Wert muss die Spannung zwischen a und b ändern damit die Gesamtstromstärke pq.A beträgt? abcliste center tikzpicture drawthick ------; drawthick --; drawthick --; drawthick ----; drawthick ---.---.; drawthick -.---.----; drawthick -.------; drawthick -----.---.--; drawthick -------.; drawthick ----; nodeabove at - a; nodeabove at b; drawthick -. rectangle -.; drawthick . rectangle .; drawthick . rectangle .; drawthick -. rectangle -.; drawthick -. rectangle .; drawthick -. rectangle -.; node at R_; node at R_; node at -. R_; node at R_; node at - R_; node at - R_; tikzpicture center
Solution:
abcliste abc Der Ersatzwiderstand des oberen Stranges ist R_uparrow R_ + R_ pq.Omega . Der Ersatzwiderstand der Parallelwiderstände im unteren Strang ist R_downarrow parallel fracfracR_+fracR_ pq.Omega . Zusammen mit R_ macht das den unteren Strang R_downarrow R_ + R_downarrow parallel pq.Omega . Der untere und obere Strang sind parallel geschaltet ihr Ersatzwiderstand des rechten Teils ist damit R_rightarrow fracfracR_uparrow+fracR_downarrow pq.Omega . Zusammen mit dem Widerstand R_ in Serie gibt das dann RersR_+R_rightarrow pq.Omega. Das sind rund pqOmega. abc Über das Ohm'sche Gesetz können wir die totale Stromstärke ausrechnen die bei pqV durch die Schaltung fliesst I fracURers pq.A . Dieser Strom fliesst auch durch R_ also I_I. abc Falls nur pqA Totalstrom fliessen so liegen gemäss Ohm'schem Gesetz nur pqV an URers I. abcliste
Contained in these collections:
-
Gleichstrom-Sudoku light by TeXercises

