Wasserstoffatom
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

Need help? Yes, please!
The following quantities appear in the problem:
Masse \(m\) / elektrische Ladung \(q, Q\) / Kraft \(F\) / Geschwindigkeit \(v\) / Strecke \(s\) / Radius \(r\) /
The following formulas must be used to solve the exercise:
\(F = \frac{1}{4\pi\epsilon_0}\cdot \frac{q_1q_2}{r^2} \quad \) \(F = m\dfrac{v^2}{r} \quad \)

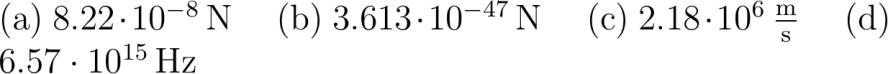
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
In einem sehr einfachen Modell vom Wasserstoffatom enquotekreist ein Elektron in einem bestimmten Abstand Bohr'scher Radius .m um den Atomkern Proton. abcliste abc Wie gross ist beim Wasserstoffatom die elektrische Anziehungskraft zwischen dem Kern und dem Elektron? abc Wie gross ist die Gravitationsanziehung zwischen den beiden Teilchen? In welchem Zahlenverhältnis stehen die beiden Kräfte? abc Mit welcher Geschwindigkeit müsste dieses Elektron um den Kern kreisen damit die Coulombkraft gerade die notwige Zentripetalbeschleunigung ergibt? abc Wie viele Umläufe müsste das Elektron in einer Sekunde durchführen? abcliste
Solution:
newqtyr.m % abclist abc solqtyFcfracpiepsilon_ fracq^r^/*pi*ncepsn*ncen**/rn**N al FC Fcf fracpiepsilon_ fracqtynce^qtyr^ FcIII abc solqtyFgG fracsscmesscmpr^ncGn*ncmen*ncmpn/rn**N al FG Fgf ncG fracncme ncmpqtyr^ Fg Das Verhältnis der Kräfte ist solnumetfracq^piepsilon_ G sscmesscmpFcn/Fgn al eta fracFCFG etf fracFcFg etIII. Die Coulombkraft ist also Grössenordnungen stärker als die Gravitationskraft! abc Die Geschwindigkeit lässt sich durch die Kreisbedingung berechnen. KreisSchritte PGleichungFC sscme aZ PGleichungfracpiepsilon_ fracq^r^ fracsscmev^r AlgebraSchritte MGleichungq^ piepsilon_ sscmerv^ MGleichungpiepsilon_ sscmerv^ q^ MGleichungv^ fracq^piepsilon_ sscmer PHYSMATH Ziehen wir die Wurzel davon und setzen die Zahlen ein erhalten wir solqtyvsqrtfracq^piepsilon_ sscmersqrtncen**/*pi*ncepsn*men*rn al v vf sqrtfracqtynce^pinceps me r vIII. abc Die Winkelgeschwindigkeit des Elektrons beträgt solqtywsqrtfracq^piepsilon_ sscmer^vn/rnrps al omega fracvr wf fracvr w. Seine Frequenz ist damit solqtyfsqrtfracq^pi^epsilon_ sscmer^wn/*piHz al f fracomegapi ff fracwpi fIII. abclist
In einem sehr einfachen Modell vom Wasserstoffatom enquotekreist ein Elektron in einem bestimmten Abstand Bohr'scher Radius .m um den Atomkern Proton. abcliste abc Wie gross ist beim Wasserstoffatom die elektrische Anziehungskraft zwischen dem Kern und dem Elektron? abc Wie gross ist die Gravitationsanziehung zwischen den beiden Teilchen? In welchem Zahlenverhältnis stehen die beiden Kräfte? abc Mit welcher Geschwindigkeit müsste dieses Elektron um den Kern kreisen damit die Coulombkraft gerade die notwige Zentripetalbeschleunigung ergibt? abc Wie viele Umläufe müsste das Elektron in einer Sekunde durchführen? abcliste
Solution:
newqtyr.m % abclist abc solqtyFcfracpiepsilon_ fracq^r^/*pi*ncepsn*ncen**/rn**N al FC Fcf fracpiepsilon_ fracqtynce^qtyr^ FcIII abc solqtyFgG fracsscmesscmpr^ncGn*ncmen*ncmpn/rn**N al FG Fgf ncG fracncme ncmpqtyr^ Fg Das Verhältnis der Kräfte ist solnumetfracq^piepsilon_ G sscmesscmpFcn/Fgn al eta fracFCFG etf fracFcFg etIII. Die Coulombkraft ist also Grössenordnungen stärker als die Gravitationskraft! abc Die Geschwindigkeit lässt sich durch die Kreisbedingung berechnen. KreisSchritte PGleichungFC sscme aZ PGleichungfracpiepsilon_ fracq^r^ fracsscmev^r AlgebraSchritte MGleichungq^ piepsilon_ sscmerv^ MGleichungpiepsilon_ sscmerv^ q^ MGleichungv^ fracq^piepsilon_ sscmer PHYSMATH Ziehen wir die Wurzel davon und setzen die Zahlen ein erhalten wir solqtyvsqrtfracq^piepsilon_ sscmersqrtncen**/*pi*ncepsn*men*rn al v vf sqrtfracqtynce^pinceps me r vIII. abc Die Winkelgeschwindigkeit des Elektrons beträgt solqtywsqrtfracq^piepsilon_ sscmer^vn/rnrps al omega fracvr wf fracvr w. Seine Frequenz ist damit solqtyfsqrtfracq^pi^epsilon_ sscmer^wn/*piHz al f fracomegapi ff fracwpi fIII. abclist
Meta Information
Exercise:
In einem sehr einfachen Modell vom Wasserstoffatom enquotekreist ein Elektron in einem bestimmten Abstand Bohr'scher Radius .m um den Atomkern Proton. abcliste abc Wie gross ist beim Wasserstoffatom die elektrische Anziehungskraft zwischen dem Kern und dem Elektron? abc Wie gross ist die Gravitationsanziehung zwischen den beiden Teilchen? In welchem Zahlenverhältnis stehen die beiden Kräfte? abc Mit welcher Geschwindigkeit müsste dieses Elektron um den Kern kreisen damit die Coulombkraft gerade die notwige Zentripetalbeschleunigung ergibt? abc Wie viele Umläufe müsste das Elektron in einer Sekunde durchführen? abcliste
Solution:
newqtyr.m % abclist abc solqtyFcfracpiepsilon_ fracq^r^/*pi*ncepsn*ncen**/rn**N al FC Fcf fracpiepsilon_ fracqtynce^qtyr^ FcIII abc solqtyFgG fracsscmesscmpr^ncGn*ncmen*ncmpn/rn**N al FG Fgf ncG fracncme ncmpqtyr^ Fg Das Verhältnis der Kräfte ist solnumetfracq^piepsilon_ G sscmesscmpFcn/Fgn al eta fracFCFG etf fracFcFg etIII. Die Coulombkraft ist also Grössenordnungen stärker als die Gravitationskraft! abc Die Geschwindigkeit lässt sich durch die Kreisbedingung berechnen. KreisSchritte PGleichungFC sscme aZ PGleichungfracpiepsilon_ fracq^r^ fracsscmev^r AlgebraSchritte MGleichungq^ piepsilon_ sscmerv^ MGleichungpiepsilon_ sscmerv^ q^ MGleichungv^ fracq^piepsilon_ sscmer PHYSMATH Ziehen wir die Wurzel davon und setzen die Zahlen ein erhalten wir solqtyvsqrtfracq^piepsilon_ sscmersqrtncen**/*pi*ncepsn*men*rn al v vf sqrtfracqtynce^pinceps me r vIII. abc Die Winkelgeschwindigkeit des Elektrons beträgt solqtywsqrtfracq^piepsilon_ sscmer^vn/rnrps al omega fracvr wf fracvr w. Seine Frequenz ist damit solqtyfsqrtfracq^pi^epsilon_ sscmer^wn/*piHz al f fracomegapi ff fracwpi fIII. abclist
In einem sehr einfachen Modell vom Wasserstoffatom enquotekreist ein Elektron in einem bestimmten Abstand Bohr'scher Radius .m um den Atomkern Proton. abcliste abc Wie gross ist beim Wasserstoffatom die elektrische Anziehungskraft zwischen dem Kern und dem Elektron? abc Wie gross ist die Gravitationsanziehung zwischen den beiden Teilchen? In welchem Zahlenverhältnis stehen die beiden Kräfte? abc Mit welcher Geschwindigkeit müsste dieses Elektron um den Kern kreisen damit die Coulombkraft gerade die notwige Zentripetalbeschleunigung ergibt? abc Wie viele Umläufe müsste das Elektron in einer Sekunde durchführen? abcliste
Solution:
newqtyr.m % abclist abc solqtyFcfracpiepsilon_ fracq^r^/*pi*ncepsn*ncen**/rn**N al FC Fcf fracpiepsilon_ fracqtynce^qtyr^ FcIII abc solqtyFgG fracsscmesscmpr^ncGn*ncmen*ncmpn/rn**N al FG Fgf ncG fracncme ncmpqtyr^ Fg Das Verhältnis der Kräfte ist solnumetfracq^piepsilon_ G sscmesscmpFcn/Fgn al eta fracFCFG etf fracFcFg etIII. Die Coulombkraft ist also Grössenordnungen stärker als die Gravitationskraft! abc Die Geschwindigkeit lässt sich durch die Kreisbedingung berechnen. KreisSchritte PGleichungFC sscme aZ PGleichungfracpiepsilon_ fracq^r^ fracsscmev^r AlgebraSchritte MGleichungq^ piepsilon_ sscmerv^ MGleichungpiepsilon_ sscmerv^ q^ MGleichungv^ fracq^piepsilon_ sscmer PHYSMATH Ziehen wir die Wurzel davon und setzen die Zahlen ein erhalten wir solqtyvsqrtfracq^piepsilon_ sscmersqrtncen**/*pi*ncepsn*men*rn al v vf sqrtfracqtynce^pinceps me r vIII. abc Die Winkelgeschwindigkeit des Elektrons beträgt solqtywsqrtfracq^piepsilon_ sscmer^vn/rnrps al omega fracvr wf fracvr w. Seine Frequenz ist damit solqtyfsqrtfracq^pi^epsilon_ sscmer^wn/*piHz al f fracomegapi ff fracwpi fIII. abclist
Contained in these collections:
-
Elektrische Kraft by pw
-
Coulomb-Kraft und Zentripetalkraft by TeXercises

