Billiards collision at right angle
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)
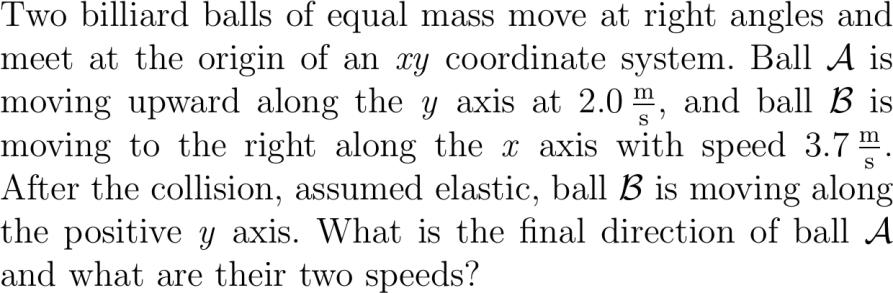
Need help? Yes, please!
The following quantities appear in the problem:
Masse \(m\) / Geschwindigkeit \(v\) / Winkel \(\theta\) / Impuls \(p\) /
The following formulas must be used to solve the exercise:
\(\cos\alpha = \dfrac{b}{c} \quad \) \(p = mv \quad \) \(\sum p_{\scriptscriptstyle\rm tot} \stackrel{!}{=} \sum p_{\scriptscriptstyle\rm tot}' \quad \) \(\sin\alpha = \dfrac{a}{c} \quad \)
No explanation / solution video for this exercise has yet been created.
But there is a video to a similar exercise:
In case your browser prevents YouTube embedding: https://youtu.be/IhqncCB0Evg
But there is a video to a similar exercise:
Exercise:
Two billiard balls of equal mass move at right angles and meet at the origin of an xy coordinate system. Ball mathcalA is moving upward along the y axis at .meterpersecond and ball mathcalB is moving to the right along the x axis with speed .meterpersecond. After the collision ased elastic ball mathcalB is moving along the positive y axis. What is the final direction of ball mathcalA and what are their two speeds?
Solution:
First we make a sketch of the situation. The left drawing shows the situation before the collision the right one the situation after the collision. center tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel-.red; Billardkugel-.blue; drawthick-green!!black -.---. nodeabovegreen!!black w; drawthick-green!!black -.---. nodeleftgreen!!black v; tikzpicture tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel..red; Billardkugel..blue; drawthick-green!!black .--. nodeleftgreen!!black v'; drawthick-green!!black :.cm -- +:.cm nodeabovegreen!!black w'; % drawthick colorred .--.---.--.; tikzpicture center We denote the momenta of the balls mathcal A and mathcal B by vec p and vec q respectively and their velocities by vec v and vec w respectively. Conservation of momentum in x- and y-direction leads to the following s: alptot &mustbe ptot' vec p + vec q vec p' + vec q' mmqtyv_xv_y + mmqtyw_xw_y mmqtyv_x'v_y' + mmqtyw_x'w_y' mqtyv + mqtyw mqtyv'cosalphav'sinalpha + mqtyw' Furthermore we have conversation of the kinetic energy: al Etot &mustbe Etot Ekin Ekin fracmv^ + frac mw^ fracmv'^ + fracmw'^ v^ + w^ v'^ + w'^. This is a system of three non-linear s with three unknowns v' w' and alpha v and w are given in the text: w v'cosalpha labelfirst v v'sinalpha + w' labelsecond v^ + w^ v'^ + w'^ labelthird Since we do not have a general procedure to solve such a system we need some creativity. A good trick for such a system is to use the fact that sin^alpha + cos^alpha i.e. we should rearrange the s in a way that we get the squares of sin and cos. We can do this by simply squaring eqreffirst and squaring eqrefsecond after taking w' to the left side: al w^ v'^cos^alpha qtyv-w'^ v'^sin^alpha We can now add these two s: al w^ + qtyv-w'^ v'^ w^ + v^ - vw' + w'^ v'^ v^ + w^ v'^-w'^ + vw' We can eliminate v' by subtracting this from eqrefthird and then solve for w': al w'^ - vw' w' v .. Putting w'v o eqrefthird and solving for v' yields al v^ + w^ v'^ + v^ v' w .. We use eqreffirst to determine alpha and thus the direction of the first ball: al alpha arccosfracwv' arccosfrac.. degree.
Two billiard balls of equal mass move at right angles and meet at the origin of an xy coordinate system. Ball mathcalA is moving upward along the y axis at .meterpersecond and ball mathcalB is moving to the right along the x axis with speed .meterpersecond. After the collision ased elastic ball mathcalB is moving along the positive y axis. What is the final direction of ball mathcalA and what are their two speeds?
Solution:
First we make a sketch of the situation. The left drawing shows the situation before the collision the right one the situation after the collision. center tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel-.red; Billardkugel-.blue; drawthick-green!!black -.---. nodeabovegreen!!black w; drawthick-green!!black -.---. nodeleftgreen!!black v; tikzpicture tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel..red; Billardkugel..blue; drawthick-green!!black .--. nodeleftgreen!!black v'; drawthick-green!!black :.cm -- +:.cm nodeabovegreen!!black w'; % drawthick colorred .--.---.--.; tikzpicture center We denote the momenta of the balls mathcal A and mathcal B by vec p and vec q respectively and their velocities by vec v and vec w respectively. Conservation of momentum in x- and y-direction leads to the following s: alptot &mustbe ptot' vec p + vec q vec p' + vec q' mmqtyv_xv_y + mmqtyw_xw_y mmqtyv_x'v_y' + mmqtyw_x'w_y' mqtyv + mqtyw mqtyv'cosalphav'sinalpha + mqtyw' Furthermore we have conversation of the kinetic energy: al Etot &mustbe Etot Ekin Ekin fracmv^ + frac mw^ fracmv'^ + fracmw'^ v^ + w^ v'^ + w'^. This is a system of three non-linear s with three unknowns v' w' and alpha v and w are given in the text: w v'cosalpha labelfirst v v'sinalpha + w' labelsecond v^ + w^ v'^ + w'^ labelthird Since we do not have a general procedure to solve such a system we need some creativity. A good trick for such a system is to use the fact that sin^alpha + cos^alpha i.e. we should rearrange the s in a way that we get the squares of sin and cos. We can do this by simply squaring eqreffirst and squaring eqrefsecond after taking w' to the left side: al w^ v'^cos^alpha qtyv-w'^ v'^sin^alpha We can now add these two s: al w^ + qtyv-w'^ v'^ w^ + v^ - vw' + w'^ v'^ v^ + w^ v'^-w'^ + vw' We can eliminate v' by subtracting this from eqrefthird and then solve for w': al w'^ - vw' w' v .. Putting w'v o eqrefthird and solving for v' yields al v^ + w^ v'^ + v^ v' w .. We use eqreffirst to determine alpha and thus the direction of the first ball: al alpha arccosfracwv' arccosfrac.. degree.
Meta Information
Exercise:
Two billiard balls of equal mass move at right angles and meet at the origin of an xy coordinate system. Ball mathcalA is moving upward along the y axis at .meterpersecond and ball mathcalB is moving to the right along the x axis with speed .meterpersecond. After the collision ased elastic ball mathcalB is moving along the positive y axis. What is the final direction of ball mathcalA and what are their two speeds?
Solution:
First we make a sketch of the situation. The left drawing shows the situation before the collision the right one the situation after the collision. center tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel-.red; Billardkugel-.blue; drawthick-green!!black -.---. nodeabovegreen!!black w; drawthick-green!!black -.---. nodeleftgreen!!black v; tikzpicture tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel..red; Billardkugel..blue; drawthick-green!!black .--. nodeleftgreen!!black v'; drawthick-green!!black :.cm -- +:.cm nodeabovegreen!!black w'; % drawthick colorred .--.---.--.; tikzpicture center We denote the momenta of the balls mathcal A and mathcal B by vec p and vec q respectively and their velocities by vec v and vec w respectively. Conservation of momentum in x- and y-direction leads to the following s: alptot &mustbe ptot' vec p + vec q vec p' + vec q' mmqtyv_xv_y + mmqtyw_xw_y mmqtyv_x'v_y' + mmqtyw_x'w_y' mqtyv + mqtyw mqtyv'cosalphav'sinalpha + mqtyw' Furthermore we have conversation of the kinetic energy: al Etot &mustbe Etot Ekin Ekin fracmv^ + frac mw^ fracmv'^ + fracmw'^ v^ + w^ v'^ + w'^. This is a system of three non-linear s with three unknowns v' w' and alpha v and w are given in the text: w v'cosalpha labelfirst v v'sinalpha + w' labelsecond v^ + w^ v'^ + w'^ labelthird Since we do not have a general procedure to solve such a system we need some creativity. A good trick for such a system is to use the fact that sin^alpha + cos^alpha i.e. we should rearrange the s in a way that we get the squares of sin and cos. We can do this by simply squaring eqreffirst and squaring eqrefsecond after taking w' to the left side: al w^ v'^cos^alpha qtyv-w'^ v'^sin^alpha We can now add these two s: al w^ + qtyv-w'^ v'^ w^ + v^ - vw' + w'^ v'^ v^ + w^ v'^-w'^ + vw' We can eliminate v' by subtracting this from eqrefthird and then solve for w': al w'^ - vw' w' v .. Putting w'v o eqrefthird and solving for v' yields al v^ + w^ v'^ + v^ v' w .. We use eqreffirst to determine alpha and thus the direction of the first ball: al alpha arccosfracwv' arccosfrac.. degree.
Two billiard balls of equal mass move at right angles and meet at the origin of an xy coordinate system. Ball mathcalA is moving upward along the y axis at .meterpersecond and ball mathcalB is moving to the right along the x axis with speed .meterpersecond. After the collision ased elastic ball mathcalB is moving along the positive y axis. What is the final direction of ball mathcalA and what are their two speeds?
Solution:
First we make a sketch of the situation. The left drawing shows the situation before the collision the right one the situation after the collision. center tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel-.red; Billardkugel-.blue; drawthick-green!!black -.---. nodeabovegreen!!black w; drawthick-green!!black -.---. nodeleftgreen!!black v; tikzpicture tikzpicturescale. latex drawdotted ---; drawdotted ---; Billardkugel..red; Billardkugel..blue; drawthick-green!!black .--. nodeleftgreen!!black v'; drawthick-green!!black :.cm -- +:.cm nodeabovegreen!!black w'; % drawthick colorred .--.---.--.; tikzpicture center We denote the momenta of the balls mathcal A and mathcal B by vec p and vec q respectively and their velocities by vec v and vec w respectively. Conservation of momentum in x- and y-direction leads to the following s: alptot &mustbe ptot' vec p + vec q vec p' + vec q' mmqtyv_xv_y + mmqtyw_xw_y mmqtyv_x'v_y' + mmqtyw_x'w_y' mqtyv + mqtyw mqtyv'cosalphav'sinalpha + mqtyw' Furthermore we have conversation of the kinetic energy: al Etot &mustbe Etot Ekin Ekin fracmv^ + frac mw^ fracmv'^ + fracmw'^ v^ + w^ v'^ + w'^. This is a system of three non-linear s with three unknowns v' w' and alpha v and w are given in the text: w v'cosalpha labelfirst v v'sinalpha + w' labelsecond v^ + w^ v'^ + w'^ labelthird Since we do not have a general procedure to solve such a system we need some creativity. A good trick for such a system is to use the fact that sin^alpha + cos^alpha i.e. we should rearrange the s in a way that we get the squares of sin and cos. We can do this by simply squaring eqreffirst and squaring eqrefsecond after taking w' to the left side: al w^ v'^cos^alpha qtyv-w'^ v'^sin^alpha We can now add these two s: al w^ + qtyv-w'^ v'^ w^ + v^ - vw' + w'^ v'^ v^ + w^ v'^-w'^ + vw' We can eliminate v' by subtracting this from eqrefthird and then solve for w': al w'^ - vw' w' v .. Putting w'v o eqrefthird and solving for v' yields al v^ + w^ v'^ + v^ v' w .. We use eqreffirst to determine alpha and thus the direction of the first ball: al alpha arccosfracwv' arccosfrac.. degree.
Contained in these collections:
-
Elastischer Stoss by pw
-
Elastischer Stoss by uz
-
Zusammenprall zwei Billardkugeln by TeXercises

