Schraubenfeder
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

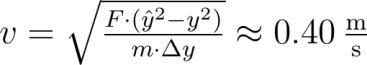
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
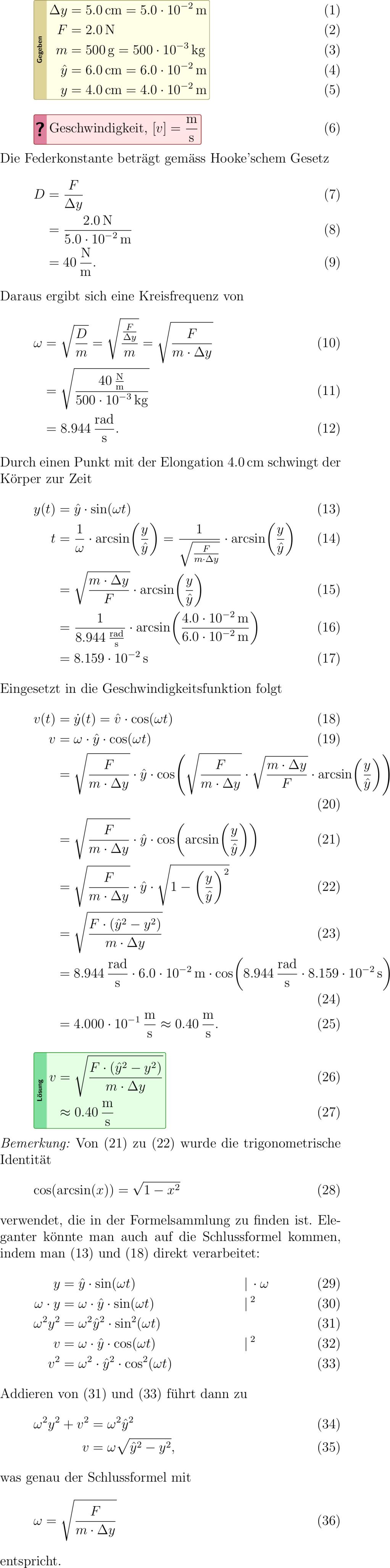
Eine Schraubenfeder erfährt eine Verlängerung von Dyo wenn sie mit F belastet wird. Sie trägt am freien Ende einen Körper von mo und wird in Schwingungen von hyo Amplitude versetzt. Wie gross ist die Geschwindigkeit des Körpers wenn er durch den Punkt mit der Elongation yo schwingt?
Solution:
Geg Delta y Dyo Dy F F m mo m hat y hyo hy y yo y % GesGeschwindigkeitv si Die Federkonstante beträgt gemäss Hooke'schem Gesetz al D Df fracFDy DTT. Daraus ergibt sich eine Kreisfrequenz von al omega sqrtfracDm sqrtfracDfm Wf sqrtfracDTTm W. Durch einen Punkt mit der Elongation yo schwingt der Körper zur Zeit al yt hat y sinomega t labely t fracomegaarcsinfracyhat y fracWfarcsinfracyhat yprv & tf fracWarcsinfracyhy t Eingesetzt in die Geschwindigkeitsfunktion folgt al vt dot yt hat v cosomega t labelv v omega hat y cosomega t prv Wf hat y cosWf tf prv Wf hat y cosarcsinfracyhat y labelcosarcsin Wf hat y sqrt-qtyfracyhat y^ labelsqrt prv vf W hy cosW t v approx vTT. % v vf &approx vTT Bemerkung: Von eqrefcosarcsin zu eqrefsqrt wurde die trigonometrische Identität al cosarcsinx sqrt-x^ verwet die in der Formelsammlung zu finden ist. Eleganter könnte man auch auf die Schlussformel kommen indem man eqrefy und eqrefv direkt verarbeitet: al y hat y sinomega t uf omega omega y omega hat y sinomega t uf ^ omega^ y^ omega^ hat y^ sinomega tlabely v omega hat y cosomega t uf ^ v^ omega^ hat y^ cosomega tlabelv Addieren von eqrefy und eqrefv führt dann zu al omega^ y^ + v^ omega^ hat y^ v omega sqrthat y^-y^ was genau der Schlussformel mit al omega Wf entspricht.
Eine Schraubenfeder erfährt eine Verlängerung von Dyo wenn sie mit F belastet wird. Sie trägt am freien Ende einen Körper von mo und wird in Schwingungen von hyo Amplitude versetzt. Wie gross ist die Geschwindigkeit des Körpers wenn er durch den Punkt mit der Elongation yo schwingt?
Solution:
Geg Delta y Dyo Dy F F m mo m hat y hyo hy y yo y % GesGeschwindigkeitv si Die Federkonstante beträgt gemäss Hooke'schem Gesetz al D Df fracFDy DTT. Daraus ergibt sich eine Kreisfrequenz von al omega sqrtfracDm sqrtfracDfm Wf sqrtfracDTTm W. Durch einen Punkt mit der Elongation yo schwingt der Körper zur Zeit al yt hat y sinomega t labely t fracomegaarcsinfracyhat y fracWfarcsinfracyhat yprv & tf fracWarcsinfracyhy t Eingesetzt in die Geschwindigkeitsfunktion folgt al vt dot yt hat v cosomega t labelv v omega hat y cosomega t prv Wf hat y cosWf tf prv Wf hat y cosarcsinfracyhat y labelcosarcsin Wf hat y sqrt-qtyfracyhat y^ labelsqrt prv vf W hy cosW t v approx vTT. % v vf &approx vTT Bemerkung: Von eqrefcosarcsin zu eqrefsqrt wurde die trigonometrische Identität al cosarcsinx sqrt-x^ verwet die in der Formelsammlung zu finden ist. Eleganter könnte man auch auf die Schlussformel kommen indem man eqrefy und eqrefv direkt verarbeitet: al y hat y sinomega t uf omega omega y omega hat y sinomega t uf ^ omega^ y^ omega^ hat y^ sinomega tlabely v omega hat y cosomega t uf ^ v^ omega^ hat y^ cosomega tlabelv Addieren von eqrefy und eqrefv führt dann zu al omega^ y^ + v^ omega^ hat y^ v omega sqrthat y^-y^ was genau der Schlussformel mit al omega Wf entspricht.
Meta Information
Exercise:
Eine Schraubenfeder erfährt eine Verlängerung von Dyo wenn sie mit F belastet wird. Sie trägt am freien Ende einen Körper von mo und wird in Schwingungen von hyo Amplitude versetzt. Wie gross ist die Geschwindigkeit des Körpers wenn er durch den Punkt mit der Elongation yo schwingt?
Solution:
Geg Delta y Dyo Dy F F m mo m hat y hyo hy y yo y % GesGeschwindigkeitv si Die Federkonstante beträgt gemäss Hooke'schem Gesetz al D Df fracFDy DTT. Daraus ergibt sich eine Kreisfrequenz von al omega sqrtfracDm sqrtfracDfm Wf sqrtfracDTTm W. Durch einen Punkt mit der Elongation yo schwingt der Körper zur Zeit al yt hat y sinomega t labely t fracomegaarcsinfracyhat y fracWfarcsinfracyhat yprv & tf fracWarcsinfracyhy t Eingesetzt in die Geschwindigkeitsfunktion folgt al vt dot yt hat v cosomega t labelv v omega hat y cosomega t prv Wf hat y cosWf tf prv Wf hat y cosarcsinfracyhat y labelcosarcsin Wf hat y sqrt-qtyfracyhat y^ labelsqrt prv vf W hy cosW t v approx vTT. % v vf &approx vTT Bemerkung: Von eqrefcosarcsin zu eqrefsqrt wurde die trigonometrische Identität al cosarcsinx sqrt-x^ verwet die in der Formelsammlung zu finden ist. Eleganter könnte man auch auf die Schlussformel kommen indem man eqrefy und eqrefv direkt verarbeitet: al y hat y sinomega t uf omega omega y omega hat y sinomega t uf ^ omega^ y^ omega^ hat y^ sinomega tlabely v omega hat y cosomega t uf ^ v^ omega^ hat y^ cosomega tlabelv Addieren von eqrefy und eqrefv führt dann zu al omega^ y^ + v^ omega^ hat y^ v omega sqrthat y^-y^ was genau der Schlussformel mit al omega Wf entspricht.
Eine Schraubenfeder erfährt eine Verlängerung von Dyo wenn sie mit F belastet wird. Sie trägt am freien Ende einen Körper von mo und wird in Schwingungen von hyo Amplitude versetzt. Wie gross ist die Geschwindigkeit des Körpers wenn er durch den Punkt mit der Elongation yo schwingt?
Solution:
Geg Delta y Dyo Dy F F m mo m hat y hyo hy y yo y % GesGeschwindigkeitv si Die Federkonstante beträgt gemäss Hooke'schem Gesetz al D Df fracFDy DTT. Daraus ergibt sich eine Kreisfrequenz von al omega sqrtfracDm sqrtfracDfm Wf sqrtfracDTTm W. Durch einen Punkt mit der Elongation yo schwingt der Körper zur Zeit al yt hat y sinomega t labely t fracomegaarcsinfracyhat y fracWfarcsinfracyhat yprv & tf fracWarcsinfracyhy t Eingesetzt in die Geschwindigkeitsfunktion folgt al vt dot yt hat v cosomega t labelv v omega hat y cosomega t prv Wf hat y cosWf tf prv Wf hat y cosarcsinfracyhat y labelcosarcsin Wf hat y sqrt-qtyfracyhat y^ labelsqrt prv vf W hy cosW t v approx vTT. % v vf &approx vTT Bemerkung: Von eqrefcosarcsin zu eqrefsqrt wurde die trigonometrische Identität al cosarcsinx sqrt-x^ verwet die in der Formelsammlung zu finden ist. Eleganter könnte man auch auf die Schlussformel kommen indem man eqrefy und eqrefv direkt verarbeitet: al y hat y sinomega t uf omega omega y omega hat y sinomega t uf ^ omega^ y^ omega^ hat y^ sinomega tlabely v omega hat y cosomega t uf ^ v^ omega^ hat y^ cosomega tlabelv Addieren von eqrefy und eqrefv führt dann zu al omega^ y^ + v^ omega^ hat y^ v omega sqrthat y^-y^ was genau der Schlussformel mit al omega Wf entspricht.
Contained in these collections:
-
-
Federpendel by pw
-
Harmonische Schwingung 0 by uz

