Ballistik: Projektil
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
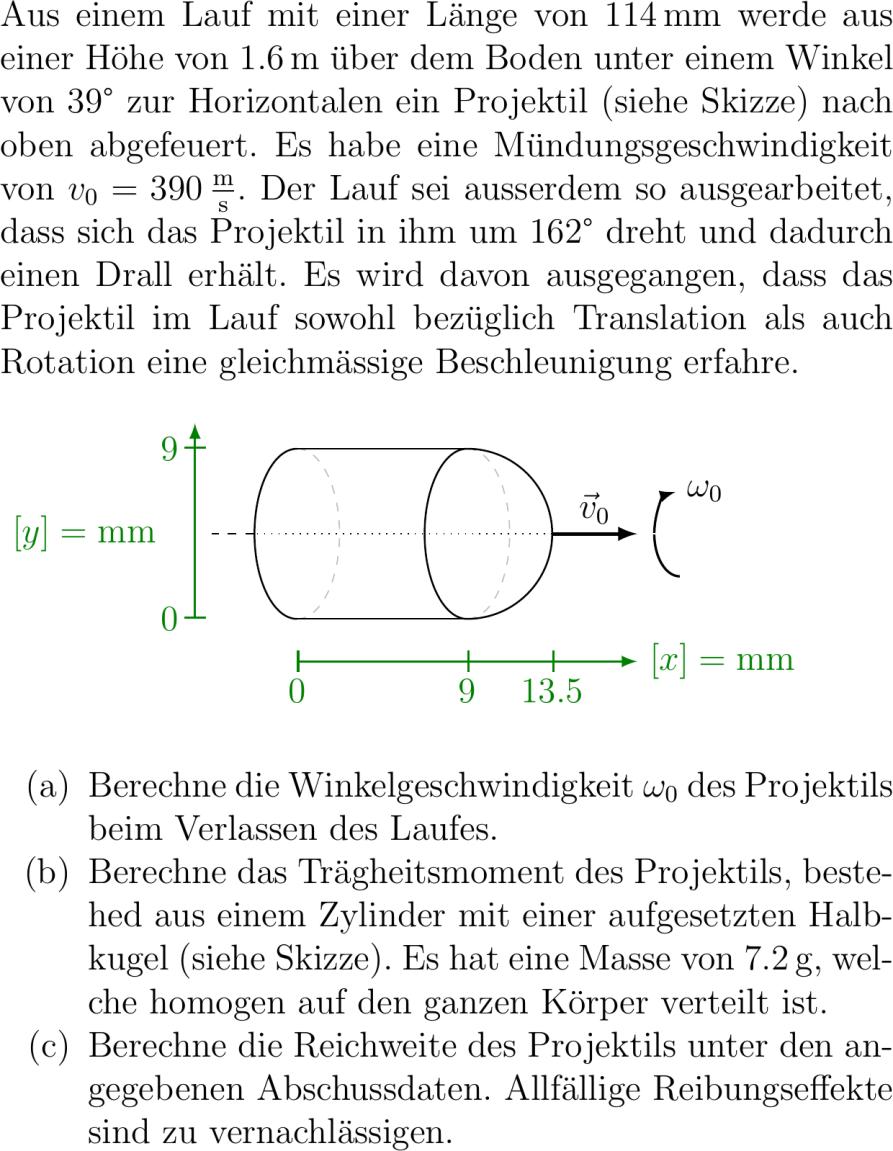
Aus einem Lauf mit einer Länge von mm werde aus einer Höhe von .m über dem Boden unter einem Winkel von ang zur Horizontalen ein Projektil siehe Skizze nach oben abgefeuert. Es habe eine Mün-dungs-gschwin-dig-keit von v_meterpersecond. Der Lauf sei ausserdem so ausgearbeitet dass sich das Projektil in ihm um ang dreht und dadurch einen Drall erhält. Es wird davon ausgegangen dass das Projektil im Lauf sowohl bezüglich Translation als auch Rotation eine gleichmässige Beschleunigung erfahre. center tikzpicturelatex %pfahl drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick -- ;% bottom line drawsemithick - -- -;% top line drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick - arc -:: and ; %drehachse drawdashed --; drawdashed -.---; drawdotted ---.; % koordinatenkreuz % drawdotted ---; % drawdotted ---; % drawdotted -.--.; % drawdotted .--.; % mündungsgeschwindigkeit drawvery thick -latex --; node at .. vec v_; % winkelgeschwindigkeit drawthick- .-. arc ::. and .; node at .. omega_; %beschriftung x-achse drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; defx-. nodecolorgreen!!black at x ; nodecolorgreen!!black at x ; nodecolorgreen!!black at x .; nodecolorgreen!!black at -. xmboxmm; %beschriftung y-achse defx-. drawthick colorgreen!!black |- x---x; drawthick colorgreen!!black |- x--x.; defx-. nodecolorgreen!!black at x- ; nodecolorgreen!!black at x ; nodecolorgreen!!black at -. ymboxmm; %skizze nach links verschieben drawcolorwhite --; tikzpicture center abcliste abc Berechne die Winkelgeschwindigkeit omega_ des Projektils beim Verlassen des Laufes. abc Berechne das Trägheitsmoment des Projektils bestehed aus einem Zylinder mit einer aufgesetzten Halbkugel siehe Skizze. Es hat eine Masse von .g welche homogen auf den ganzen Körper verteilt ist. abc Berechne die Reichweite des Projektils unter den angegebenen Abschussdaten. Allfällige Reibungseffekte sind zu vernachlässigen. abcliste
Solution:
abcliste itema Die Zeit welche sich das Geschoss im Lauf befindet ist gegeben durch: t fracsv_ .s Daraus kann man die Winkelgeschwindigkeit errechnen: omega_ fracgammat .eradianpersecond abc Das Volumen des Projektils ist: V V_Z + V_K pi r^ h + frac frac pi r^ pi r^ + frac pi r^ Daraus ergibt sich die Verteilung der Masse .g auf die beiden Teilkörper: m m_Z + m_K .g + .g .g Somit kann nun das Trägheitsmoment berechnet werden: I I_Z + I_K frac m_Z r^ + frac frac m_K r^ .kgm^ + .kgm^ .kgm^ abc Die Flugzeit des Geschosses wird durch seine vertikale Bewegung bestimmt. Sie ist gegeben durch die folge quadratische Gleichung: frac-gt^ + v_uparrow t + s_ frac-gt^ + v_ sinbeta t + s_ Diese Gleichung hat die folgen Lösungen: t_ -.s t_ .s Nur die zweite ist physikalisch sinnvoll. Währ dieser Zeit fliegt das Geschoss horizontal mit -- wenn Reibung vernachlässigt wird -- unveränderter Geschwindigkeit weiter. Dadurch ergibt sich die folge Reichweite: s_x v_rightarrow t v_ cosbeta t m abcliste
Aus einem Lauf mit einer Länge von mm werde aus einer Höhe von .m über dem Boden unter einem Winkel von ang zur Horizontalen ein Projektil siehe Skizze nach oben abgefeuert. Es habe eine Mün-dungs-gschwin-dig-keit von v_meterpersecond. Der Lauf sei ausserdem so ausgearbeitet dass sich das Projektil in ihm um ang dreht und dadurch einen Drall erhält. Es wird davon ausgegangen dass das Projektil im Lauf sowohl bezüglich Translation als auch Rotation eine gleichmässige Beschleunigung erfahre. center tikzpicturelatex %pfahl drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick -- ;% bottom line drawsemithick - -- -;% top line drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick - arc -:: and ; %drehachse drawdashed --; drawdashed -.---; drawdotted ---.; % koordinatenkreuz % drawdotted ---; % drawdotted ---; % drawdotted -.--.; % drawdotted .--.; % mündungsgeschwindigkeit drawvery thick -latex --; node at .. vec v_; % winkelgeschwindigkeit drawthick- .-. arc ::. and .; node at .. omega_; %beschriftung x-achse drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; defx-. nodecolorgreen!!black at x ; nodecolorgreen!!black at x ; nodecolorgreen!!black at x .; nodecolorgreen!!black at -. xmboxmm; %beschriftung y-achse defx-. drawthick colorgreen!!black |- x---x; drawthick colorgreen!!black |- x--x.; defx-. nodecolorgreen!!black at x- ; nodecolorgreen!!black at x ; nodecolorgreen!!black at -. ymboxmm; %skizze nach links verschieben drawcolorwhite --; tikzpicture center abcliste abc Berechne die Winkelgeschwindigkeit omega_ des Projektils beim Verlassen des Laufes. abc Berechne das Trägheitsmoment des Projektils bestehed aus einem Zylinder mit einer aufgesetzten Halbkugel siehe Skizze. Es hat eine Masse von .g welche homogen auf den ganzen Körper verteilt ist. abc Berechne die Reichweite des Projektils unter den angegebenen Abschussdaten. Allfällige Reibungseffekte sind zu vernachlässigen. abcliste
Solution:
abcliste itema Die Zeit welche sich das Geschoss im Lauf befindet ist gegeben durch: t fracsv_ .s Daraus kann man die Winkelgeschwindigkeit errechnen: omega_ fracgammat .eradianpersecond abc Das Volumen des Projektils ist: V V_Z + V_K pi r^ h + frac frac pi r^ pi r^ + frac pi r^ Daraus ergibt sich die Verteilung der Masse .g auf die beiden Teilkörper: m m_Z + m_K .g + .g .g Somit kann nun das Trägheitsmoment berechnet werden: I I_Z + I_K frac m_Z r^ + frac frac m_K r^ .kgm^ + .kgm^ .kgm^ abc Die Flugzeit des Geschosses wird durch seine vertikale Bewegung bestimmt. Sie ist gegeben durch die folge quadratische Gleichung: frac-gt^ + v_uparrow t + s_ frac-gt^ + v_ sinbeta t + s_ Diese Gleichung hat die folgen Lösungen: t_ -.s t_ .s Nur die zweite ist physikalisch sinnvoll. Währ dieser Zeit fliegt das Geschoss horizontal mit -- wenn Reibung vernachlässigt wird -- unveränderter Geschwindigkeit weiter. Dadurch ergibt sich die folge Reichweite: s_x v_rightarrow t v_ cosbeta t m abcliste
Meta Information
Exercise:
Aus einem Lauf mit einer Länge von mm werde aus einer Höhe von .m über dem Boden unter einem Winkel von ang zur Horizontalen ein Projektil siehe Skizze nach oben abgefeuert. Es habe eine Mün-dungs-gschwin-dig-keit von v_meterpersecond. Der Lauf sei ausserdem so ausgearbeitet dass sich das Projektil in ihm um ang dreht und dadurch einen Drall erhält. Es wird davon ausgegangen dass das Projektil im Lauf sowohl bezüglich Translation als auch Rotation eine gleichmässige Beschleunigung erfahre. center tikzpicturelatex %pfahl drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick -- ;% bottom line drawsemithick - -- -;% top line drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick - arc -:: and ; %drehachse drawdashed --; drawdashed -.---; drawdotted ---.; % koordinatenkreuz % drawdotted ---; % drawdotted ---; % drawdotted -.--.; % drawdotted .--.; % mündungsgeschwindigkeit drawvery thick -latex --; node at .. vec v_; % winkelgeschwindigkeit drawthick- .-. arc ::. and .; node at .. omega_; %beschriftung x-achse drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; defx-. nodecolorgreen!!black at x ; nodecolorgreen!!black at x ; nodecolorgreen!!black at x .; nodecolorgreen!!black at -. xmboxmm; %beschriftung y-achse defx-. drawthick colorgreen!!black |- x---x; drawthick colorgreen!!black |- x--x.; defx-. nodecolorgreen!!black at x- ; nodecolorgreen!!black at x ; nodecolorgreen!!black at -. ymboxmm; %skizze nach links verschieben drawcolorwhite --; tikzpicture center abcliste abc Berechne die Winkelgeschwindigkeit omega_ des Projektils beim Verlassen des Laufes. abc Berechne das Trägheitsmoment des Projektils bestehed aus einem Zylinder mit einer aufgesetzten Halbkugel siehe Skizze. Es hat eine Masse von .g welche homogen auf den ganzen Körper verteilt ist. abc Berechne die Reichweite des Projektils unter den angegebenen Abschussdaten. Allfällige Reibungseffekte sind zu vernachlässigen. abcliste
Solution:
abcliste itema Die Zeit welche sich das Geschoss im Lauf befindet ist gegeben durch: t fracsv_ .s Daraus kann man die Winkelgeschwindigkeit errechnen: omega_ fracgammat .eradianpersecond abc Das Volumen des Projektils ist: V V_Z + V_K pi r^ h + frac frac pi r^ pi r^ + frac pi r^ Daraus ergibt sich die Verteilung der Masse .g auf die beiden Teilkörper: m m_Z + m_K .g + .g .g Somit kann nun das Trägheitsmoment berechnet werden: I I_Z + I_K frac m_Z r^ + frac frac m_K r^ .kgm^ + .kgm^ .kgm^ abc Die Flugzeit des Geschosses wird durch seine vertikale Bewegung bestimmt. Sie ist gegeben durch die folge quadratische Gleichung: frac-gt^ + v_uparrow t + s_ frac-gt^ + v_ sinbeta t + s_ Diese Gleichung hat die folgen Lösungen: t_ -.s t_ .s Nur die zweite ist physikalisch sinnvoll. Währ dieser Zeit fliegt das Geschoss horizontal mit -- wenn Reibung vernachlässigt wird -- unveränderter Geschwindigkeit weiter. Dadurch ergibt sich die folge Reichweite: s_x v_rightarrow t v_ cosbeta t m abcliste
Aus einem Lauf mit einer Länge von mm werde aus einer Höhe von .m über dem Boden unter einem Winkel von ang zur Horizontalen ein Projektil siehe Skizze nach oben abgefeuert. Es habe eine Mün-dungs-gschwin-dig-keit von v_meterpersecond. Der Lauf sei ausserdem so ausgearbeitet dass sich das Projektil in ihm um ang dreht und dadurch einen Drall erhält. Es wird davon ausgegangen dass das Projektil im Lauf sowohl bezüglich Translation als auch Rotation eine gleichmässige Beschleunigung erfahre. center tikzpicturelatex %pfahl drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick -- ;% bottom line drawsemithick - -- -;% top line drawdashedcolorgray - arc -::. and ;% right half of the left ellipse drawsemithick - arc ::. and ;% left half of the left ellipse drawsemithick - arc -:: and ; %drehachse drawdashed --; drawdashed -.---; drawdotted ---.; % koordinatenkreuz % drawdotted ---; % drawdotted ---; % drawdotted -.--.; % drawdotted .--.; % mündungsgeschwindigkeit drawvery thick -latex --; node at .. vec v_; % winkelgeschwindigkeit drawthick- .-. arc ::. and .; node at .. omega_; %beschriftung x-achse drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; drawthick colorgreen!!black |- -.---.; defx-. nodecolorgreen!!black at x ; nodecolorgreen!!black at x ; nodecolorgreen!!black at x .; nodecolorgreen!!black at -. xmboxmm; %beschriftung y-achse defx-. drawthick colorgreen!!black |- x---x; drawthick colorgreen!!black |- x--x.; defx-. nodecolorgreen!!black at x- ; nodecolorgreen!!black at x ; nodecolorgreen!!black at -. ymboxmm; %skizze nach links verschieben drawcolorwhite --; tikzpicture center abcliste abc Berechne die Winkelgeschwindigkeit omega_ des Projektils beim Verlassen des Laufes. abc Berechne das Trägheitsmoment des Projektils bestehed aus einem Zylinder mit einer aufgesetzten Halbkugel siehe Skizze. Es hat eine Masse von .g welche homogen auf den ganzen Körper verteilt ist. abc Berechne die Reichweite des Projektils unter den angegebenen Abschussdaten. Allfällige Reibungseffekte sind zu vernachlässigen. abcliste
Solution:
abcliste itema Die Zeit welche sich das Geschoss im Lauf befindet ist gegeben durch: t fracsv_ .s Daraus kann man die Winkelgeschwindigkeit errechnen: omega_ fracgammat .eradianpersecond abc Das Volumen des Projektils ist: V V_Z + V_K pi r^ h + frac frac pi r^ pi r^ + frac pi r^ Daraus ergibt sich die Verteilung der Masse .g auf die beiden Teilkörper: m m_Z + m_K .g + .g .g Somit kann nun das Trägheitsmoment berechnet werden: I I_Z + I_K frac m_Z r^ + frac frac m_K r^ .kgm^ + .kgm^ .kgm^ abc Die Flugzeit des Geschosses wird durch seine vertikale Bewegung bestimmt. Sie ist gegeben durch die folge quadratische Gleichung: frac-gt^ + v_uparrow t + s_ frac-gt^ + v_ sinbeta t + s_ Diese Gleichung hat die folgen Lösungen: t_ -.s t_ .s Nur die zweite ist physikalisch sinnvoll. Währ dieser Zeit fliegt das Geschoss horizontal mit -- wenn Reibung vernachlässigt wird -- unveränderter Geschwindigkeit weiter. Dadurch ergibt sich die folge Reichweite: s_x v_rightarrow t v_ cosbeta t m abcliste
Contained in these collections:
-
PAM Matura 2014 Stans by uz
-
Matura-Training Mechanik by uz

