Massive Kugeln
About points...
We associate a certain number of points with each exercise.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
When you click an exercise into a collection, this number will be taken as points for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit the number of points for the exercise in the collection independently, without any effect on "points by default" as represented by the number here.
That being said... How many "default points" should you associate with an exercise upon creation?
As with difficulty, there is no straight forward and generally accepted way.
But as a guideline, we tend to give as many points by default as there are mathematical steps to do in the exercise.
Again, very vague... But the number should kind of represent the "work" required.
About difficulty...
We associate a certain difficulty with each exercise.
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
When you click an exercise into a collection, this number will be taken as difficulty for the exercise, kind of "by default".
But once the exercise is on the collection, you can edit its difficulty in the collection independently, without any effect on the "difficulty by default" here.
Why we use chess pieces? Well... we like chess, we like playing around with \(\LaTeX\)-fonts, we wanted symbols that need less space than six stars in a table-column... But in your layouts, you are of course free to indicate the difficulty of the exercise the way you want.
That being said... How "difficult" is an exercise? It depends on many factors, like what was being taught etc.
In physics exercises, we try to follow this pattern:
Level 1 - One formula (one you would find in a reference book) is enough to solve the exercise. Example exercise
Level 2 - Two formulas are needed, it's possible to compute an "in-between" solution, i.e. no algebraic equation needed. Example exercise
Level 3 - "Chain-computations" like on level 2, but 3+ calculations. Still, no equations, i.e. you are not forced to solve it in an algebraic manner. Example exercise
Level 4 - Exercise needs to be solved by algebraic equations, not possible to calculate numerical "in-between" results. Example exercise
Level 5 -
Level 6 -
Question
Solution
Short
Video
\(\LaTeX\)

Need help? Yes, please!
The following quantities appear in the problem:
Masse \(m\) / Kraft \(F\) / Volumen \(V\) / Radius \(r\) / Dichte \(\varrho\) /
The following formulas must be used to solve the exercise:
\(\varrho = \dfrac{m}{V} \quad \) \(F = G \dfrac{m_1m_2}{r^2} \quad \) \(V = \dfrac{4}{3}\pi r^3 \quad \)
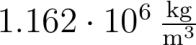
No explanation / solution video to this exercise has yet been created.
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Visit our YouTube-Channel to see solutions to other exercises.
Don't forget to subscribe to our channel, like the videos and leave comments!
Exercise:
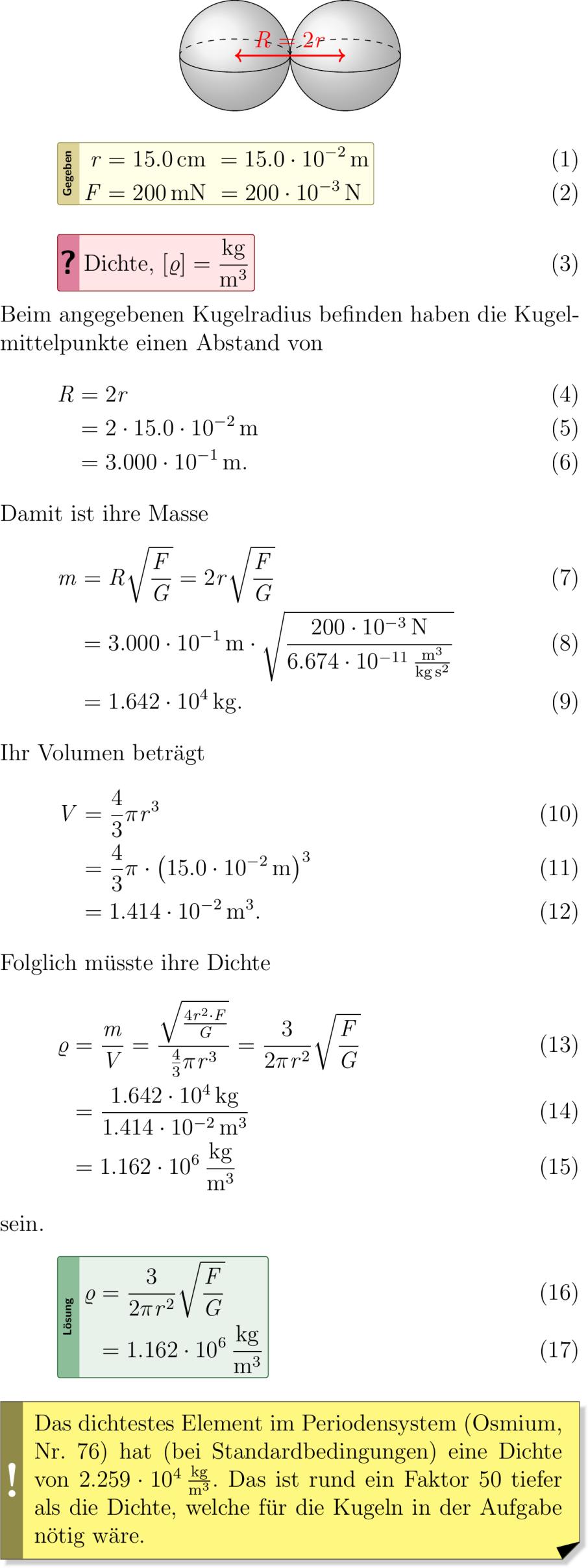
Zwei identische massive Kugeln mit je .cm Radius berühren sich. Welche Dichte müsste das Material der homogenen Kugeln aufweisen damit sie sich aufgrund der Gravitation mit mN anziehen?
Solution:
center tikzpicture shadeball color gray! opacity. - circle ; draw - circle ; draw - arc :: and .; drawdashed arc :: and .; shadeball color gray! opacity. circle ; draw circle ; draw arc :: and .; drawdashed arc :: and .; drawthick red - --- nodemidway above Rr; tikzpicture center newqtyr.m newqtyFN % Geg r .cm r F mN F % GesDichtevarrho sikgpcm % Beim angegebenen Kugelradius befinden haben die Kugelmittelpunkte einen Abstand von solqtyRr*rnm al R Rf r R. Damit ist ihre Masse solqtymrsqrtfracFG*rn*sqrtFn/Gnkg al m RsqrtfracFG mf R sqrtfracFG m. Ihr Volumen beträgt solqtyVfracpi r^/*pi*rn**cubicmeter al V Vf fracpi qtyr^ V. Folglich müsste ihre Dichte solqtypfracpi r^sqrtfracFGmn/Vnkgpcm al varrho fracmV fracsqrtfracr^ FGfrac pi r^ pf fracmV p sein. varrho pf p Ausrufbox Das dichtestes Element im Periodensystem Osmium Nr. hat bei Standardbedingungen eine Dichte von .ekgpmk. Das ist rund ein Faktor tiefer als die Dichte welche für die Kugeln in der Aufgabe nötig wäre. Ausrufbox
Zwei identische massive Kugeln mit je .cm Radius berühren sich. Welche Dichte müsste das Material der homogenen Kugeln aufweisen damit sie sich aufgrund der Gravitation mit mN anziehen?
Solution:
center tikzpicture shadeball color gray! opacity. - circle ; draw - circle ; draw - arc :: and .; drawdashed arc :: and .; shadeball color gray! opacity. circle ; draw circle ; draw arc :: and .; drawdashed arc :: and .; drawthick red - --- nodemidway above Rr; tikzpicture center newqtyr.m newqtyFN % Geg r .cm r F mN F % GesDichtevarrho sikgpcm % Beim angegebenen Kugelradius befinden haben die Kugelmittelpunkte einen Abstand von solqtyRr*rnm al R Rf r R. Damit ist ihre Masse solqtymrsqrtfracFG*rn*sqrtFn/Gnkg al m RsqrtfracFG mf R sqrtfracFG m. Ihr Volumen beträgt solqtyVfracpi r^/*pi*rn**cubicmeter al V Vf fracpi qtyr^ V. Folglich müsste ihre Dichte solqtypfracpi r^sqrtfracFGmn/Vnkgpcm al varrho fracmV fracsqrtfracr^ FGfrac pi r^ pf fracmV p sein. varrho pf p Ausrufbox Das dichtestes Element im Periodensystem Osmium Nr. hat bei Standardbedingungen eine Dichte von .ekgpmk. Das ist rund ein Faktor tiefer als die Dichte welche für die Kugeln in der Aufgabe nötig wäre. Ausrufbox
Meta Information
Exercise:
Zwei identische massive Kugeln mit je .cm Radius berühren sich. Welche Dichte müsste das Material der homogenen Kugeln aufweisen damit sie sich aufgrund der Gravitation mit mN anziehen?
Solution:
center tikzpicture shadeball color gray! opacity. - circle ; draw - circle ; draw - arc :: and .; drawdashed arc :: and .; shadeball color gray! opacity. circle ; draw circle ; draw arc :: and .; drawdashed arc :: and .; drawthick red - --- nodemidway above Rr; tikzpicture center newqtyr.m newqtyFN % Geg r .cm r F mN F % GesDichtevarrho sikgpcm % Beim angegebenen Kugelradius befinden haben die Kugelmittelpunkte einen Abstand von solqtyRr*rnm al R Rf r R. Damit ist ihre Masse solqtymrsqrtfracFG*rn*sqrtFn/Gnkg al m RsqrtfracFG mf R sqrtfracFG m. Ihr Volumen beträgt solqtyVfracpi r^/*pi*rn**cubicmeter al V Vf fracpi qtyr^ V. Folglich müsste ihre Dichte solqtypfracpi r^sqrtfracFGmn/Vnkgpcm al varrho fracmV fracsqrtfracr^ FGfrac pi r^ pf fracmV p sein. varrho pf p Ausrufbox Das dichtestes Element im Periodensystem Osmium Nr. hat bei Standardbedingungen eine Dichte von .ekgpmk. Das ist rund ein Faktor tiefer als die Dichte welche für die Kugeln in der Aufgabe nötig wäre. Ausrufbox
Zwei identische massive Kugeln mit je .cm Radius berühren sich. Welche Dichte müsste das Material der homogenen Kugeln aufweisen damit sie sich aufgrund der Gravitation mit mN anziehen?
Solution:
center tikzpicture shadeball color gray! opacity. - circle ; draw - circle ; draw - arc :: and .; drawdashed arc :: and .; shadeball color gray! opacity. circle ; draw circle ; draw arc :: and .; drawdashed arc :: and .; drawthick red - --- nodemidway above Rr; tikzpicture center newqtyr.m newqtyFN % Geg r .cm r F mN F % GesDichtevarrho sikgpcm % Beim angegebenen Kugelradius befinden haben die Kugelmittelpunkte einen Abstand von solqtyRr*rnm al R Rf r R. Damit ist ihre Masse solqtymrsqrtfracFG*rn*sqrtFn/Gnkg al m RsqrtfracFG mf R sqrtfracFG m. Ihr Volumen beträgt solqtyVfracpi r^/*pi*rn**cubicmeter al V Vf fracpi qtyr^ V. Folglich müsste ihre Dichte solqtypfracpi r^sqrtfracFGmn/Vnkgpcm al varrho fracmV fracsqrtfracr^ FGfrac pi r^ pf fracmV p sein. varrho pf p Ausrufbox Das dichtestes Element im Periodensystem Osmium Nr. hat bei Standardbedingungen eine Dichte von .ekgpmk. Das ist rund ein Faktor tiefer als die Dichte welche für die Kugeln in der Aufgabe nötig wäre. Ausrufbox
Contained in these collections:
-
Gravitation by aej
-
Gravitationsgesetz by pw
-
-
Zwei Kugeln by TeXercises
Physical Quantity
Massendichte
Verhältnis von Masse zu Volumen
\(\varrho = \dfrac{m}{V}\)
Unit
Kilogramm pro Kubikmeter (\(\rm \frac{kg}{m^3}\))
Base?
SI?
Metric?
Coherent?
Imperial?

